The 3 Body Problem
El problema de los tres cuerpos
(With Albert Merino)

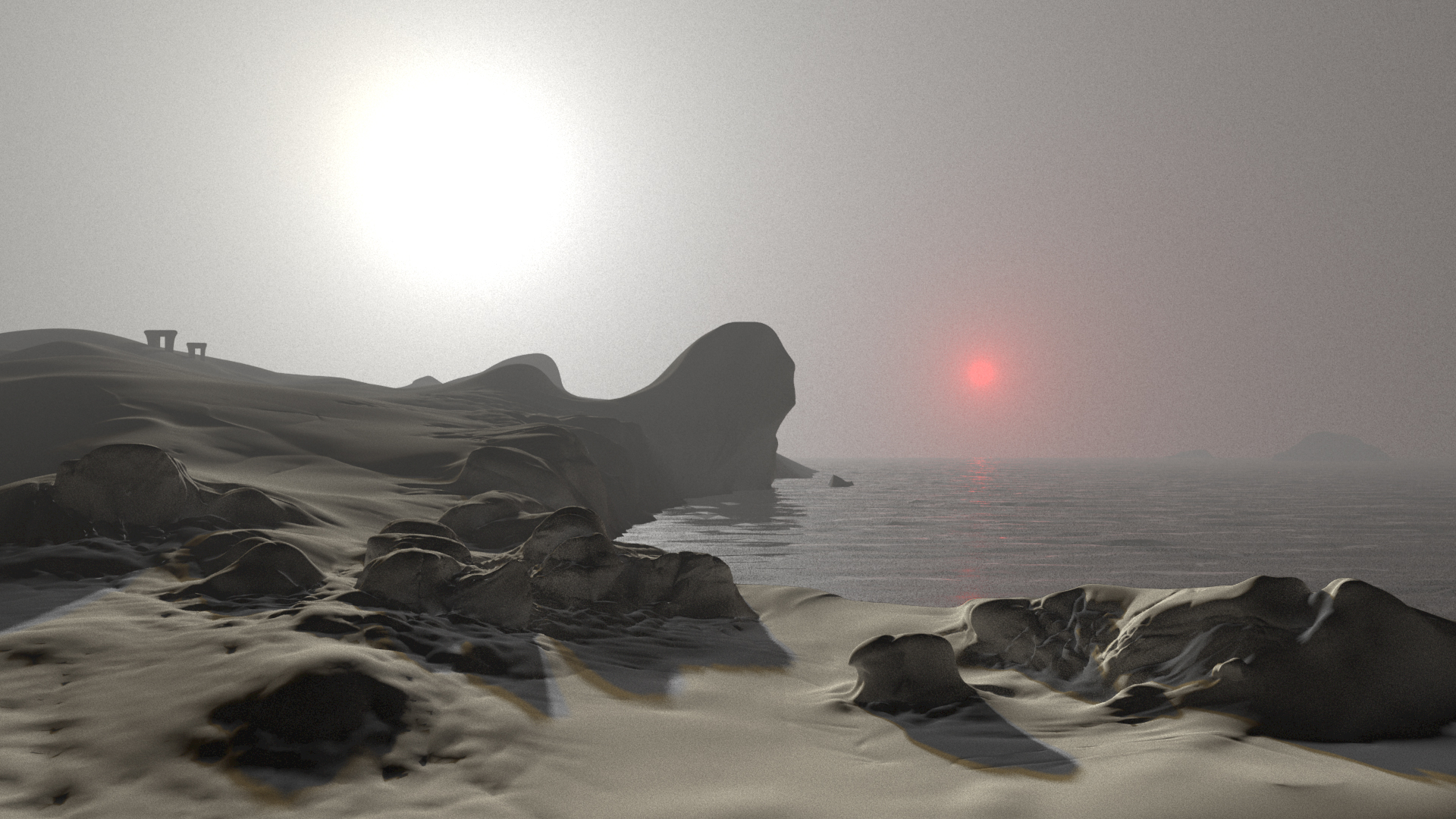
When visiting the Museum of Modern Art of Tarragona to prepare the exhibition offered by the Biennal d'Art de la Diputació de Tarragona, I noticed a painting belonging to its collection entitled The Sphinx of Roscoff. Painted in 1910 by José Nogué, it shows a sunrise on a deserted beach whose rocks suggest the silhouette of a sphinx. Since the classical Greek sphinx is a symbiosis of three bodies; head of a woman, wings of a bird and body of a feline, it seemed pertinent to make an adaptation of the painting that would show a triple sunrise.

"The problem of the three bodies" is a mathematical problem that appears just after Kepler in the seventeenth century proposed elliptical solutions for the movements of gravitational attraction between celestial bodies. Kepler offers a very simple way to calculate how two bodies are moving when they are attracted to each other, but he realizes that if his formula is applied to systems of more than two stars (which is not uncommon in the universe; the closest star, Alpha Centauri, is a trisolar system) it becomes very difficult to deduce their trajectories. The movements become a chaotic dance, and the system turns to be unstable, tending to expel one of its members or collapse over time, as happens with love triangles.

With the help of Albert Merino, we have created a three-dimensional map of the landscape painted by Nogué, and we have projected onto a copy of the painting an accelerated animation of three suns orbiting each other, which move based on examples of calculations of the "three-body problem" downloaded from scientific pages. Each sun belongs to a different type of star and emits light in a different color. The multiple sunrises and sunsets of different suns and their orbital dances produce changes in the atmosphere of the painting, tinting the air and the sea with colors and altering the shadows of the painted stones.

Visitando el Museo de Arte Moderno de Tarragona para preparar la exposición ofrecida por la Biennal d'Art de la Diputació de Tarragona, me fijé en un cuadro perteneciente a su colección titulado La Esfinge de Roscoff y pintado el 1910 por José Nogué que muestra un amanecer en una playa desierta cuyas rocas dejan adivinar la silueta de una esfinge. Como la esfinge clásica es una simbiosis de tres cuerpos; cabeza de mujer, alas de pájaro y cuerpo de felino, me pareció pertinente hacer una adaptación del cuadro que mostrara una salida de sol triple.

"El problema de los tres cuerpos" es un problema matemático que aparece justo después de que Kepler en el siglo XVII proponga soluciones elípticas para los movimientos de atracción gravitatoria entre cuerpos celestes. Kepler ofrece una forma muy sencilla para calcular cómo dos cuerpos se mueven atraídos entre ellos, pero se da cuenta de que si su fórmula se aplica a sistemas de más de dos estrellas (algo que en el universo no es infrecuente; la estrella más cercana, Alfa Centauri, es un sistema trisolar) se hace muy difícil deducir sus trayectorias. Los movimientos se vuelven una danza caótica, y el sistema se hace inestable, tendiendo a expulsar a uno de sus miembros o a colapsar al cabo de tiempo, como ocurre con los triángulos amorosos.

Con Albert Merino, hemos levantado un mapa tridimensional del paisaje pintado por Nogué, y hemos proyectado sobre una copia del cuadro una animación acelerada de tres soles orbitando entre ellos, que se mueven a partir de ejemplos de cálculos del "problema de los tres cuerpos" bajados de páginas científicas. Cada sol pertenece a un tipo diferente de estrella y emite luz en distinto color. Las múltiples salidas y puestas de soles distintos y sus danzas orbitales producen cambios en la atmósfera del cuadro, tiñendo de colores el aire y el mar y alterando las sombras de las piedras pintadas.

Videoinstallation
3d animation projected over oil painting · 4 x 2 m · 2022 · 20 min in loop
In collaboration with Albert Merino

Produced by The Modern Art Museum of Tarragona.
Adquired by The Caixaforum Foundation.

Shown at:
"The 3 Body Problem" Modern Art Museum of Tarragona. July - September 2022
"Horizon and limit" Caixaforum Madrid. November 2023 - March 2024
"Horizon and limit" Caixaforum Barcelona. June 2024 - September 2024
"Horizon and limit" Caixaforum Sevilla. December 2024 - March 2025

Original oil painting "The Sphinx of Roscoff" by José Nogué 1910.
Collection of The Museum of Modern Art of Tarragona.

The missing panel.

The painter José Nogué

Copy of "The Sphinx of Roscoff" to use as a screen

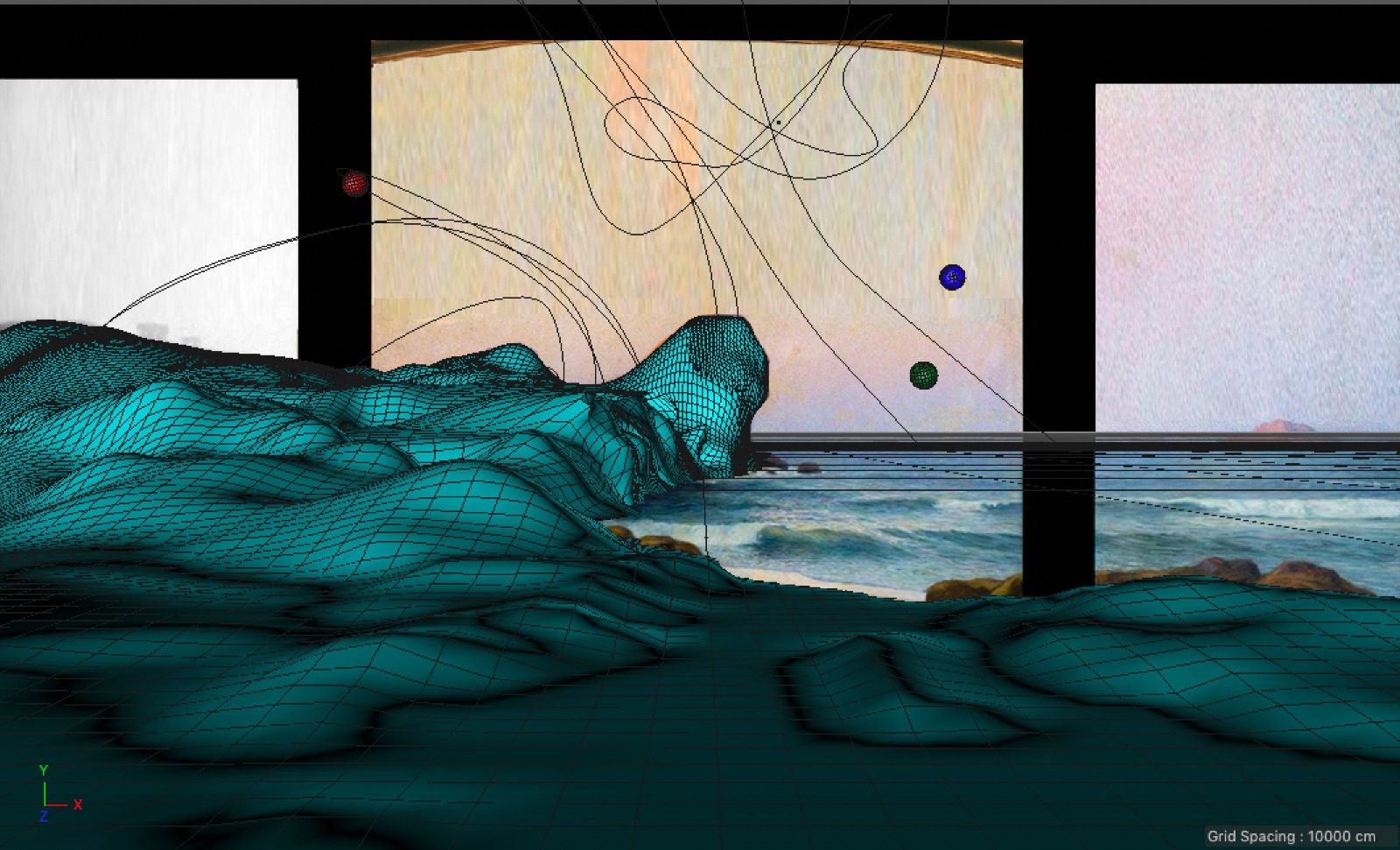
3D Animation process. Created by Albert Merino.